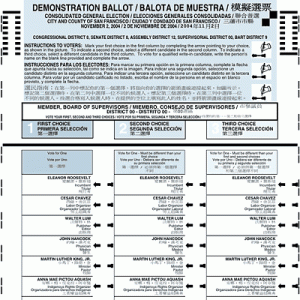
First off, a quick preview of what the new ballot looks like. A good mock-up is available at San Francisco’s Department of Election’s site. The basic format is fairly simple: Three side-by-side columns (first-choice, second-choice and third-choice). Each column includes the names of all 16 candidates.
Round 1: An Even Starting Line
From the pool of 16 candidates, you pick your first, second, and third choices. (Note: you don’t have to pick three; if you want, you can just pick your first-choice, or your first two choices, etc. It also doesn’t do you any good to repeatedly pick your first-choice three times – it’ll only be counted once.
If any candidate gets more than 50 percent (50% +1) of first-choice votes, that candidate is automatically elected. Game over. But, if no one receives that majority, we go to the second round.
So just for kicks (and because puppets are more fun than politicians), let’s pretend we’re observing a heated mayoral race on Sesame Street (Just ignore that streets don’t typically have mayors, or the fact that the candidates are made of felt and fur.). There are four candidates running, and a total of 24 voters casting ballots.
Cookie Monster (the clear frontrunner, of course, well loved for his oratorical gifts and promises of free pastries to every supporter) gets 10 first-place votes. Oscar the Grouch gets 8 first place votes (with strong support from the waste management industry and a large contingent of the generally disgruntled). Big Bird gets 4 first-place votes. And poor, impetuous Grover gets only 2 first-place votes. Since no one got more than 12 votes, there’s no clear majority, but we do have our first loser … so we move on to Round 2.
Round 2: The First Elimination
Grover, the candidate with the least amount of first-choice votes, is outta there! But (and here’s the part that seems to trip people up the most), for the two voters who picked Grover as a first-choice, their second-choice votes still count. Here’s how:
One of the voters who chose Grover picked Oscar as a second choice. So that vote goes to Oscar (who now has a total of 9 votes). The other voter in Grover’s small fan club picked Cookie Monster as a second choice. So, that vote goes to Cookie Monster (who now has 11 votes).
At the end of Round 2, here’s the tally:
Cookie Monster: 11 votes
Oscar: 9 votes
Big Bird: 4 votes
Still no clear winner (because there still are three candidates standing), so onto Round 3 we go!
Round 3: The Deciding Moment
Three candidates left, and Big Bird’s got the least amount of first-choice votes (only 4), so that oversized avian is done! Now, we look at the second-choice votes of those four voters who picked Big Bird as their first-choice. Remarkably, as it turns out, all four of Big Bird’s second-choice votes were for Oscar! That means that Oscar picks up four more votes, giving him (or it?) a final tally of 13 votes to Cookie Monster’s 11 votes. And thus, that grumpy, trash-dwelling green dude is the new boss in town.
O.K., so in the San Francisco mayoral election, things might not be quite that simple (and all the candidates are probably going to have noses). But, hopefully this example does illustrate how a candidate can viably receive the most first-choice votes and still lose the election. Because there are 16 candidates in the real race, that same elimination process keeps going until one candidate emerges with the most votes.
One key to understanding the RCV instant runoff process is remembering that the number of elimination rounds is determined by the number of candidates running. So, in the case of San Francisco’s mayoral race: there are 16 candidates, thus, 15 elimination rounds to determine a winner. In the Sesame Street scenario, there are a total of 4 candidates, requiring three elimination rounds to determine the winner. Just think of it as last man/woman/puppet standing. Take a look:
Round 1: Four candidates on the ballot with a total of 24 votes cast.
C. Monster O. Grouch B. Bird Grover Total votes
10 votes 8 votes 4 votes 2 votes 24
Round 2: Three candidates standing; Grover is eliminated and his votes go to Cookie Monster and Oscar. Remember that all 24 votes still count, but some have just been transferred to other candidates.
C. Monster O. Grouch B. Bird Total votes
11 votes 9 votes 4 votes 24
Round 3: Two candidates left; Big Bird is eliminated and all four of his votes go to Oscar (because the people who voted for Big Bird as their first-choice picked Oscar as their second-choice.
C. Monster O. Grouch Total votes
11 votes 13 votes 24
With 13 votes to Cookie Monster’s 11, Oscar the Grouch is the winner!
Oakland’s 2010 Mayoral Election
Last year, Oakland used RCV to elect its mayor and witnessed a similar outcome: There were 10 candidates, and Don Perata, the clear frontrunner (who vastly outspent his opponents during the campaign), got 35% of the first-choice votes. That left Jean Quan in a distant second with only 24% of first-choice votes. But Quan – who anticipated this outcome and allied herself with other underdog candidates and their supporters – received far more second-choice votes than did Perata. And after all the elimination rounds, with second and third-choice votes factored in, Quan received 51% of the vote to Perata’s 49%.
The Critics
So is RCV a good thing? The jury’s still out. It really depends on who you ask. (Oakland’s Mayor Quan, I’m guessing would say yes; Don Perata … not so much. Oscar the Grouch though, is definitely a big fan.)
Like pretty much everything in politics, the system’s got its strong supporters and staunch enemies.
Some of the big arguments from supporters of RCV:
- It could save taxpayers millions by eliminating the need for local primaries and separate runoff elections.
- It boosts electoral competition because candidates only have to raise money for one election per cycle, not two or three.
- It gives underdog candidates a better chance and produces a winner that’s supported by a clear majority.
- It discourages mudslinging and negative campaigning; candidates are now more likely to ally with each.
Opponents say:
- It’s too confusing for voters and unnecessarily adds to the complexity of an already complicated ballot.
- There is lots of room for technical error as election computers tally results through the use of a complicated algorithm.
- It encourages less popular candidates to game the system by teaming up against the frontrunner. Is this is a fair or appropriate strategy? Depends who you ask.
- It’s discriminatory to less educated or knowledgeable segments of the voting public who haven’t received sufficient instruction on how the system works.