Caleb: It’s like dominoes.
Christine: Yeah, you’re going to see that some patterns might remind you of dominoes. I’m going to flash it up there. Try and see how you can figure out how many are up there without counting one by one.
Christine also introduced hand signals for Number Talks, specifically putting a number of fingers at your chest to indicate how many strategies you have. A student asked, “A strategy is a different way of counting?” Christine said, “Exactly.” After flashing up the image of the dots (Figure 2.5), Christine then collected answers—how many dots students saw. They all reported seeing eight dots.
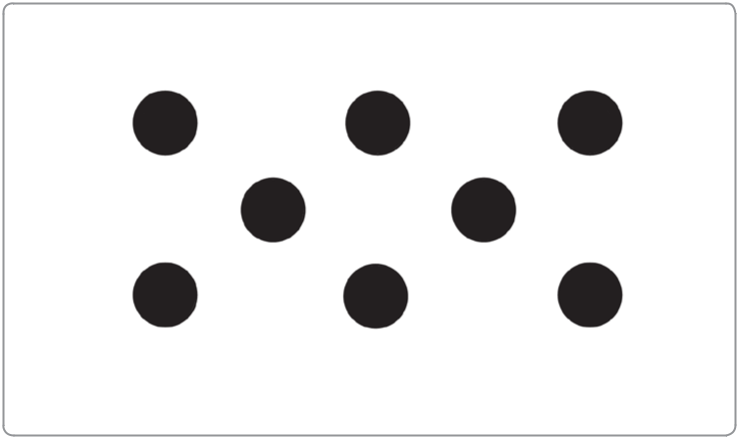
Figure 2.5 Dot Pattern for Christine’s Number Talk
Christine then invited students to share: “Can anybody be brave enough to share and justify why they think there’s eight dots up there?” A number of students went up to the whiteboard to draw how they grouped and chunked the diagram to count. The first student saw a set of five, as if it was a side of dice, plus three more. The second student saw the three on top, the three on the bottom, and the two in the middle. Another student came up to the front of the room and gestured to indicate diagonal lines:
Jason: So I saw these three and then these three. So there were six and then I made them into one sideways diamond. Then I added the two on the ends. To me this looks like a diamond.
Christine: Jason’s saying he sees a shape, is this what you’re showing? What shape is that? (Christine drew in lines as seen in Figure 2.6).
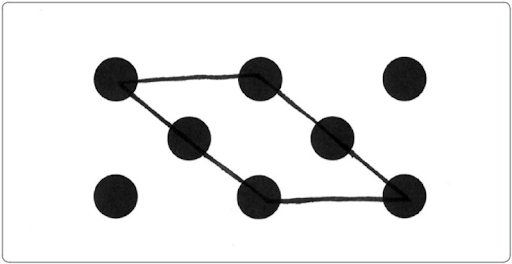
Figure 2.6 Jason sees a shape
Students brainstormed names for the shape, saying things like pentagon, parallelogram, rhombus, or “I can’t remember.” Christine identified the terms that students used and asked them to describe what they are (e.g., “What’s a pentagon?”). Then she asked, “So what can we call that shape?” Jason replied, “A weird rectangle thing.” Calista said, “It’s a parallelogram.” Another student shouted out, “It’s a thingy!” Christine said, “Calista is telling us that it’s a parallelogram.” Then she went on to say, “Yeah, we could describe it either way, but if we call it a parallelogram, that’s using more precise language that we can all visualize.” Michael Reitemeyer, the math coach in Christine’s district, was also in the room and asked, “So is it okay during rough draft talk to maybe call it a weird rectangle?” Christine turned to her students and said, “What do we think, as a class?” Students said, yes, so she said “Sure, we can accept it. Absolutely. Rough draft talk helps us.”
There were multiple moments when students engaged in rough draft sharing during this Number Talk. The first was the moment the teacher intended, which was when students shared multiple ways of seeing how to chunk the diagram to count the total. This sort of exploratory talk invites multiple ways of seeing mathematical relationships. But there was a moment that Christine did not necessarily anticipate, which was rough draft talk around how Jason named the shape he saw in the diagram. She shifted back and forth with students from rough draft to more formal language to begin refining their rough draft talk, but then affirmed that the rough draft language of “a weird rectangle” was a useful way to get started talking about the shape. Taking moments to talk about talking is important, but it is even more important to give students firsthand experience engaging in rough draft talk and to name and label those rough draft moments. …
Revise Solutions: My Favorite Rough Draft
Stefanie Vascellaro, a sixth-grade teacher at Meredith Middle School in the Appoquinimink School District in Middletown, Delaware, created an instructional routine that she called My Favorite Rough Draft.
First, Stefanie’s students work in pairs to write a rough draft of an explanation. While students are working, Stefanie circulates and considers which explanations might be fruitful to revise together. She selects one based on her mathematical and social goals for the class and then pulls the large group together to discuss it. She displays it anonymously, and the class works together to improve the explanation. She modified this routine from My Favorite No (Teaching Channel 2011), which is when a teacher chooses a common procedural error and discusses with the class what was correct about the work and where it needed to be improved. Stefanie has her students write explanations for why procedural mistakes were errors rather than explaining the error to her students herself.
As an example, she asked pairs of students to write about the following:
Read and answer the problem with your shoulder partner. Explain whether the expression on the left side of the equal sign is equivalent to the expression on the right side. Show all work.
14x^2 + 6 – 9x^2 + 4x = 9x^2 + 6
She assumed that students would combine 14x^2 and -9x^2 with 4x rather than recognize that 4x was a term that could not be combined with the other two terms. One of the goals for this task was for students to go beyond catching an error to interpreting why someone might do this. Students were expected to reflect on the meaning of “combining like terms.” Stefanie also wanted students to practice using language of the discipline of mathematics, such as combine like terms, coefficient, constant, variable, expression, and exponent.
During My Favorite Rough Draft, students’ thinking is first elicited when they talk through their initial explanations—before they write. When sixth grader Arturo talked through his ideas for his explanation with his partner, his rough draft thinking sounded like this:
Arturo: But then you have to think about it. You can’t do an exponent if you’re using—so, it’s 5x to the second power (after combining 14x^2 with -9x^2). You can’t do 5x to the second power plus 4x. It doesn’t work. Because 4x, it would have to go to the second power. You can’t add a second power to that.
You can hear the initial roughness in Arturo’s thinking when he cuts himself off (“if you’re using—”). Also, the repetitiveness in his talk is what often happens when we’re sorting out our thoughts.
After each pair talked and then wrote a draft, Stefanie had two pairs of students join to make groups of four, which is a structure she used frequently so students gain additional perspectives. The pairs swapped their drafts and edited one another’s work by suggesting revisions to their peers’ drafts. After a few minutes of editing, they got to see revisions from their peers.
When Stefanie circulated the class to choose her Favorite Rough Draft, many of the drafts were correct, so Stefanie chose a draft to revise with the class that could have included more mathematical terms. For instance, Stefanie wanted students to use terminology such as exponents. We can see in the students’ rough draft talk that they did not use that term.
Choosing a draft to revise as a class involves choosing student work that needs improving in a particular way for a particular reason. Perhaps we want students’ revisions to include more visual illustrations, to use precise vocabulary, to make their points more clearly, or to make connec-tions more explicit. But when looking for a Favorite Rough Draft, whether or not it is correct, it is useful to look for the strengths in that draft so the class can build on them. In other words, even as we select a goal for improvement, we should also notice and highlight the productive thinking in the original draft, which helps us all learn what to do similarly next time. Critique involves recognizing what is working as well as improving what is not working.
While revising the draft, Stefanie asked students to recommend changes and to give justifications for those suggestions. Not all changes are improvements! Suggestions for changes are stronger when the people making the suggestions justify why the change makes the draft stronger. In addition to revealing students’ mathematical thinking, hearing students’ justifications also provides insight as to whether students are attending to the goal for revision. Revising work as a class helps students learn to accept feedback as well.
To validate students’ thinking, Stefanie explained how revising the draft supported the group’s learning. She said that she displayed the students’ draft anonymously to protect students who may otherwise feel uncomfort-able having their work critiqued by peers. A second benefit is that an anonymous draft can help the revision process feel collectively owned by the group: the draft becomes the class’s draft, and the entire class benefits from the opportunity to think it through. If we make this explicit—the ways in which the draft helps everyone’s thinking improve—then the author of the draft is positioned as having contributed something valuable to the class.
Consider phasing out the use of anonymous drafts. After norms are in place such that in-progress thinking is seen as valuable, students can feel safer having their work publicly revised with their name attached to it. If we highlight strengths in the draft while revising, students might even feel proud of their drafts.
My Favorite Rough Draft involves multiple cycles of revision. After thinking individually, students share that initial thinking with their partners. Through cocreating a written draft with a peer, their own ideas might shift. Pairs of students then trade with another pair of students and receive feedback on their drafts that they can apply toward another revision. A further opportunity to revise thinking occurs when the class revises the Favorite Rough Draft selected by the teacher. In this particular example, Stefanie used this revision structure to analyze students’ explanations of a procedural mistake, but you could use My Favorite Rough Draft to analyze drafts of proofs, justifications, or other forms of mathematical reasoning.
*Note: due to an earlier error, exponents did not appear properly in the sample equations. This except has been updated.
